An Objection to Decision Theory?

Click here and press the right key for the next slide.

(This may not work on mobile or ipad. You can try using chrome or firefox, but even that may fail. Sorry.)

also ...

Press the left key to go backwards (or swipe right)

Press n to toggle whether notes are shown (or add '?notes' to the url before the #)

Press m or double tap to slide thumbnails (menu)

Press ? at any time to show the keyboard shortcuts

An Objection to Decision Theory?

Before we get to the objection I want to explain the larger argument I am embarking on

overview

Problem: how to characterize the goal-directed process such that we can generate action predictions.

Candidate solution: the goal-directed process is the computation of expected utility.

Objection: the goal-directed process does not compute expected utiltiy.

Illustration: Ellsberg Paradox

This is what we are doing now.

There are 90 balls in this urn.

30 of these balls are red.

The other balls are either black (all over) or yellow (all over).

One ball will be selected at random.

Please choose between:

option 1 : £100 if the ball is red, £0 otherwise

option 2 : £100 if the ball is black, £0 otherwise

Please choose between:

option A : £100 if the ball is red or yellow, £0 otherwise

option B : £100 if the ball is black or yellow, £0 otherwise

When I’ve done this in the past, most people go for option (1) over option 2.

... and they also go for option B over option A.

But this combination of options is a problem.

Can you say why it is a problem?

How to objection

1. State the construal of decision theory.

2. State the finding.

3. State the axiom it contradicts.

4. Explain how the finding contradicts the axiom.

5. (If possible, explain why it is significant.)

6. Consider responses.

identiyf the axiom it contradicts ...

Using Steele & Stefánsson (2020, p. §2.3) here.

transitivity

For any A, B, C ∈ S: if A⪯B and B⪯C then A⪯C.

(Steele & Stefánsson, 2020)

completeness

For any A, B ∈ S: either A⪯B or B⪯A

continuity

‘Continuity implies that no outcome is so bad that you would not be willing to take some gamble that might result in you ending up with that outcome [...] provided that the chance of the bad outcome is small enough.’

Suppose A⪯B⪯C. Then there is a p∈[0,1] such that: {pA, (1 − p)C} ∼ B (Steele & Stefánsson, 2020)

independence

roughly, if you prefer A to B then you should prefer A and C to B and C.

Suppose A⪯B. Then for any C, and any p∈[0,1]: {pA,(1−p)C}⪯{pB,(1−p)C}

Steele & Stefánsson (2020, p. §2.3)

complication : different formalizations

No way you are expected to understand every formalization. So you will run into great difficulty reading Ellsberg ...

‘Savage (1972, p. 21ff)'s Postulate 2, which he calls the "Sure-thing Principle"’

(Ellsberg, 1961, p. 649)

How to objection

1. State the construal of decision theory.

2. State the finding.

3. State the axiom it contradicts.

4. Explain how the finding contradicts the axiom.

5. (If possible, explain why it is significant.)

6. Consider responses.

explain how the finding contradicts the axoim ...

Not much to say here. It’s built to contradict the Independence Axiom.

[FLICK BACK AND FORWARDS THIS AND NEXT SLIDE]

There are 90 balls in this urn.

30 of these balls are red.

The other balls are either black (all over) or yellow (all over).

One ball will be selected at random.

Please choose between:

option 1 : £100 if the ball is red, £0 otherwise

option 2 : £100 if the ball is black, £0 otherwise

Please choose between:

option A : £100 if the ball is red or yellow, £0 otherwise

option B : £100 if the ball is black or yellow, £0 otherwise

Using Steele & Stefánsson (2020, p. §2.3) here.

transitivity

For any A, B, C ∈ S: if A⪯B and B⪯C then A⪯C.

(Steele & Stefánsson, 2020)

completeness

For any A, B ∈ S: either A⪯B or B⪯A

continuity

Suppose A⪯B⪯C. Then there is a p∈[0,1] such that: {pA, (1 − p)C} ∼ B (Steele & Stefánsson, 2020)

independence

roughly, if you prefer A to B then you should prefer A and C to B and C.

Suppose A⪯B. Then for any C, and any p∈[0,1]: {pA,(1−p)C}⪯{pB,(1−p)C}

Steele & Stefánsson (2020, p. §2.3)

Btw, the Independence Axiom seems quite reasonable in many contexts.

choice 1

£100 if Brazil win the world cup, £0 otherwise

£100 if France win the world cup, £0 otherwise

choice 2

£100 if Brazil or Ukraine win the world cup, £0 otherwise

£100 if France or Ukraine win the world cup, £0 otherwise

How to objection

1. State the construal of decision theory.

2. State the finding.

3. State the axiom it contradicts.

4. Explain how the finding contradicts the axiom.

5. (If possible, explain why it is significant.)

6. Consider responses.

explain significance of the contradction ...

uncertainty vs risk

Sometimes people prefer less uncertainty.

Even when less uncertainty is more risky (Jia, Furlong, Gao, Santos, & Levy, 2020) ...

What if you inform people about the Ellsberg Paradox and show how it is costing them money?

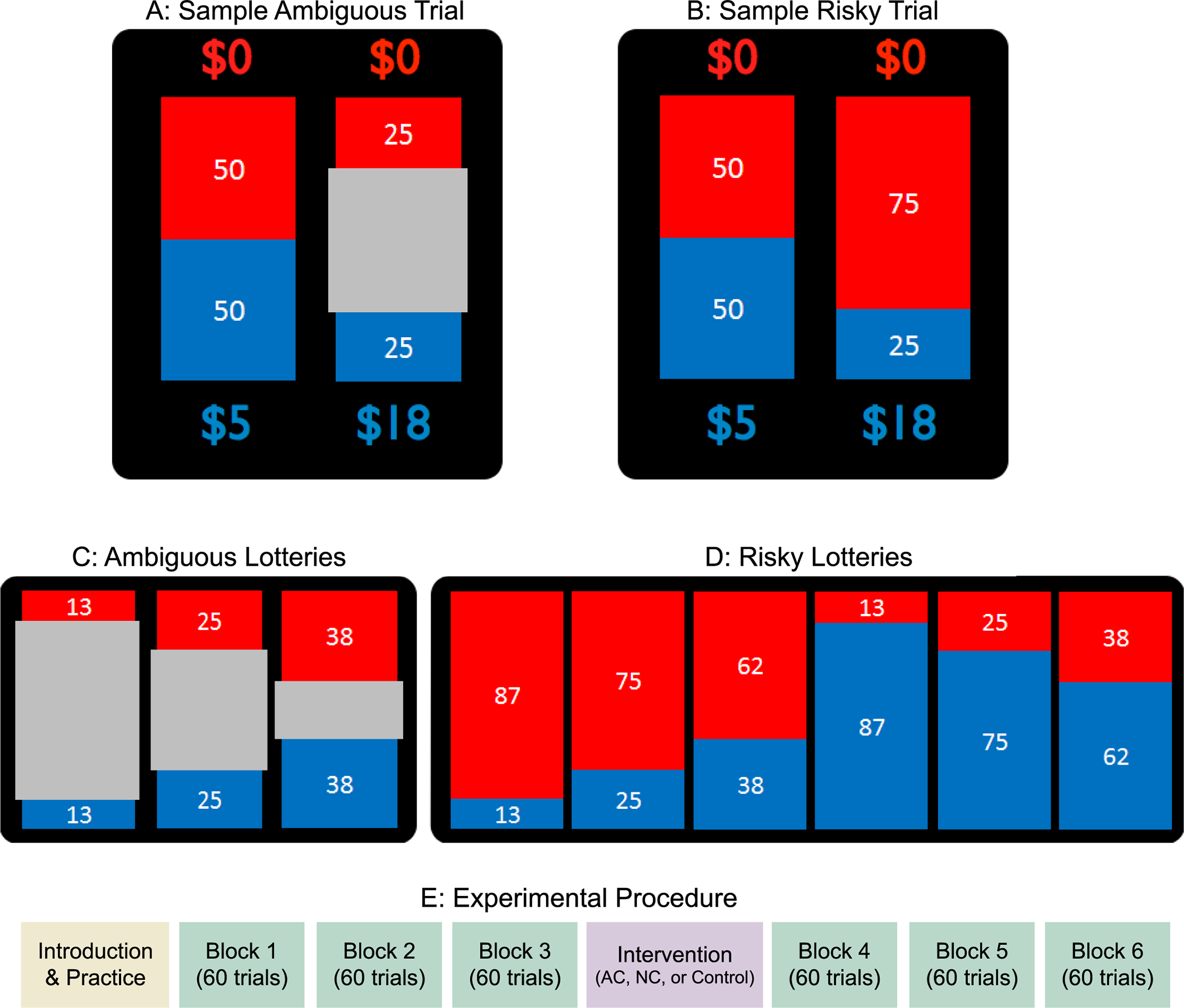
Jia et al. (2020, p. figure 1 (part))

What if you inform people about the Ellsberg Paradox and show how it is costing them money?

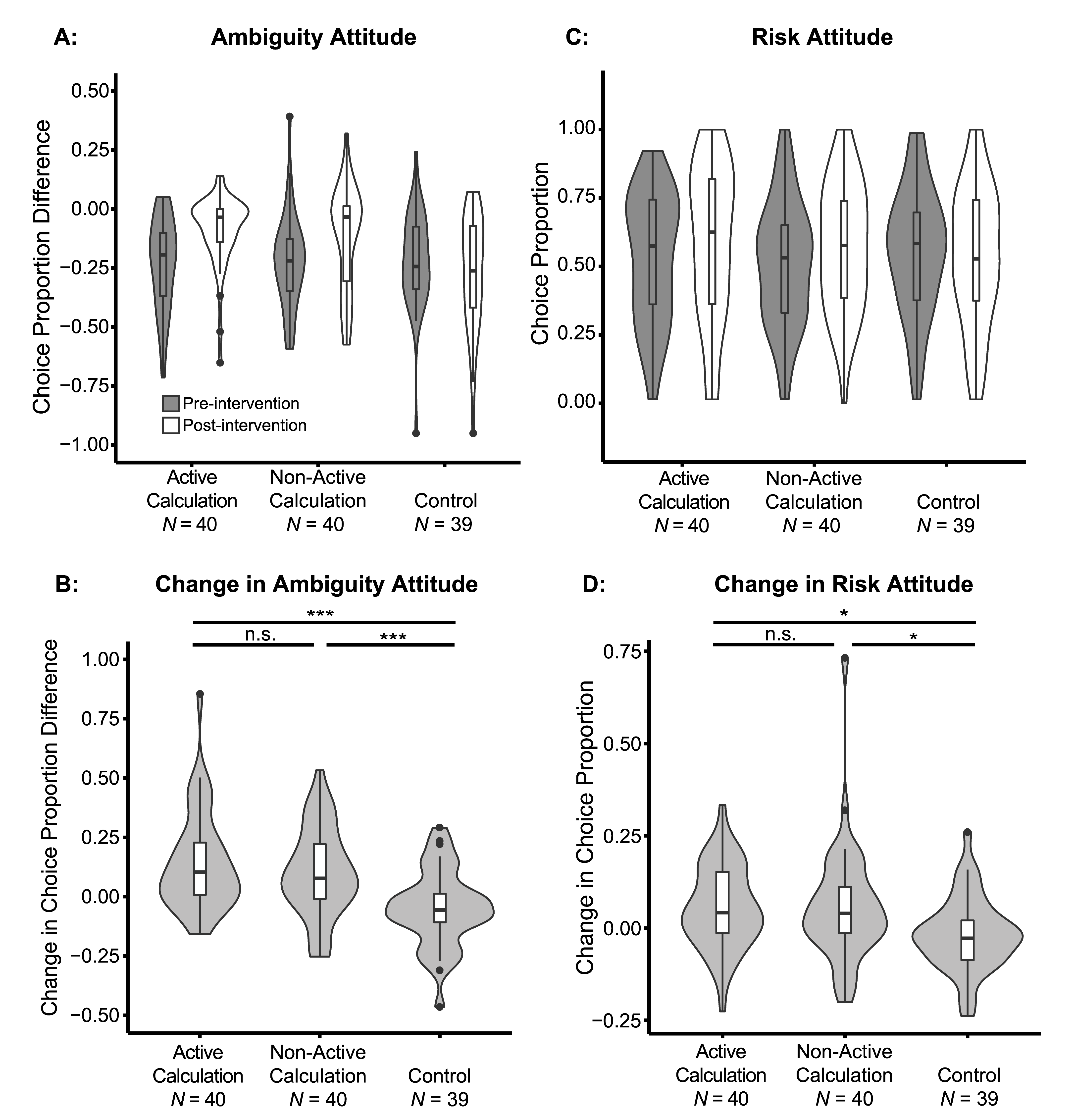
Jia et al. (2020, p. figure 4)

What if you inform people about the Ellsberg Paradox and show how it is costing them money?

‘awareness of the detrimental effect of ambiguity aversion [...] does reduce ambiguity aversion

BUT ‘ambiguity aversion [...] did not disappear

AND ‘participants also reduced their aversion to risk, suggesting inappropriate generalization of the learning to another irrelevant decision context.’

Jia et al. (2020)

How to objection

1. State the construal of decision theory.

2. State the finding.

3. State the axiom it contradicts.

4. Explain how the finding contradicts the axiom.

5. (If possible, explain why it is significant.)

6. Consider responses.

consider responses ...

Ellsberg’s own response

response 1

‘Daniel Ellsberg is an American activist and former United States military analyst who, while employed by the RAND Corporation, precipitated a national political controversy in 1971 when he released the Pentagon Papers, a top-secret Pentagon study of the US government decision-making in relation to the Vietnam War, to The New York Times and other newspapers.’ (https://www.colorado.edu/cwa/daniel-ellsberg)

‘On January 3, 1973, Ellsberg was charged under the Espionage Act of 1917 along with other charges of theft and conspiracy, carrying a total maximum sentence of 115 years. Due to governmental misconduct and illegal evidence gathering, he was dismissed of all charges on May 11, 1973.’ (https://www.colorado.edu/cwa/daniel-ellsberg)

‘both the predictive and normative use of the Savage or equivalent postulates might be improved by avoiding attempts to apply them in certain, specifiable circumstances where they do not seem acceptable’

Ellsberg (1961, p. 646)

As far as I know, Ellsberg did not explain how to do this.

Interesting that he is proposing to back of the normative use (i.e. he seems to think that aversion to ambiguity may be rational).

response 2

This suggests one line of response ...

‘we wish to find the mathematically complete principles which define “rational behavior” for the participants in a social economy, and to derive from them the general characteristics of that behavior’

(Neumann, Morgenstern, Rubinstein, & Kuhn, 1953, p. 31).

von Neumann & Morgenstern, 1953 p. 31

Is there really an objection here? Not obvious that there is any objection if we think of decision theory as a merely normative device.

‘the laws of decision theory [...] are not empirical generalisations about all agents. What they do is define what is meant ... by being rational’

(Davidson, 1987, p. 43)

But remember that we are excited about decision theory because it might clarify what we mean by belief and desire ...

In any case, there is another kind of objection that we did not consider.

This response raises two questions.

Q1 : Are people who take a less ambiguous but more risky option actually irrational?

I.e. even if we agree with Davidson about the aims, we might think that there is a faiulre to define being rational.

∞todo also relevant here is Mandler’s objection to the claim that the axioms capture rationality (commented out below): ‘Completeness applies to preference as choice, while transitivity applies to preference as a set of judgments of well-being. Convincing arguments for the axioms taken together cannot be assembled on either definition.’ (Mandler, 2001, p. 374; see also Mandler, 2005)

[leads to next section ...]

Q2 : Are there other applications of decision theory to which preferences to reduce ambiguity would be an objection?

(E.g. Jeffrey (1983, p. xi) construes decision theory as an ‘elucidation of the notions of subjective probability and subjective desirability’.)

How to objection

1. State the construal of decision theory.

2. State the finding.

3. State the axiom it contradicts.

4. Explain how the finding contradicts the axiom.

5. (If possible, explain why it is significant.)

6. Consider responses.

all done!

Consider objections carefully.

Our challenge is to understand how both of these points can be true simultaneously (amazing insights + compelling objections).

dilemma

Decision theory and game theory have generated some amazing insights (see O’Connor, 2019, for example).

This will be more of a focus when we come to game theory.

Apparently compelling objections abound.

solution

Decision theory is a model. Think carefully about how it is being construed.

How to objection

1. State the construal of decision theory.

2. State the finding.

3. State the axiom it contradicts.

4. Explain how the finding contradicts the axiom.

5. (If possible, explain why it is significant.)

6. Consider responses.

The main point for this section, though, is that handling an objection properly is a bit more involved than you might think.

It is not enough to state a finding and say that it is an objection.

Keyboard Shortcuts?f

An Objection to Decision Theory?

Keyboard Shortcuts`?f`